The general form of inhomogenous linear differential equations is:
x'' + p(t) x' + q(t) x = g(t)We need 2 initial conditions x(0) = x0, x'(0) = u0 to have a unique solution, there is a 3-step method to solve it:
- Solve the homogeneous equation, where g(t) = 0, to find the general solution to the homogeneous equation:
xh(t) = C1 x1(t) + C2 x2(t). - Find the particular solution to the inhomegenous differential equation
xp(t). - Write general solution as x(t) = xh(t) + xp(t) . Determine C1 and C2 from initial conditions.
Inhomogeneous Term
| Exponential Functions | Ansatz x = A et |
| Sine or Cosine Functions | Ansatz x = A cos(t) + B sin(t) or Leverage eit = cos(t) + i sin(t) ,convert function to complex, then use ansatz z = C eit |
| Polynomials | Ansatz x = A t2 + B t + C |

Resonance
Resonance is a phenomenon which can be observed when somebody sings against a wine glass at the glass’s frequency, causing that glass vibrates violently until it breaks. One dimensional model of resonance is below, this is a second order linear constant coefficient equation that has an inhomogeneous term:
x'' + ω02 x = f cosωtThe homogeneous part of this equation, i.e. x'' + ω02 x = 0 , is the wine glass itself. ω0 is the natural frequency. The right hand side f cosωt is modeling the voice of singer, at the frequency ω . Resonance is going to happen when ω is getting close to ω0 .
We will solve this equation when ω0 and ω are different frequencies, then we will limit the solution as ω goes to ω0.

Applications
There are some nice applications of these second order differential equations
RLC (resistor, capacitor, inductor) circuit, connected to an AC current
The constitutive relations for these circuit elements are:
- The voltage drop across a resistor is equal to the current in the circuit times the resistance.
VR = i R - The voltage drop across a capacitor is equal to the charge on the capacitor divided by the capacitance.
VC = q / C - The voltage drop across the inductor is equal to the derivative of the current with respect to time, times L.
VC = di / dt L - The current is equal to the time derivative of the charge.
i = dq / dt - The AC current can be modeled as
ε(t) = ε0 cos ωt
Differential equation comes from Kirchhoff’s law, which says the voltage supplied by the AC, is equal to the voltage drop across each elements in the circuit when they are in series ε(t) = VL +VR + VC .
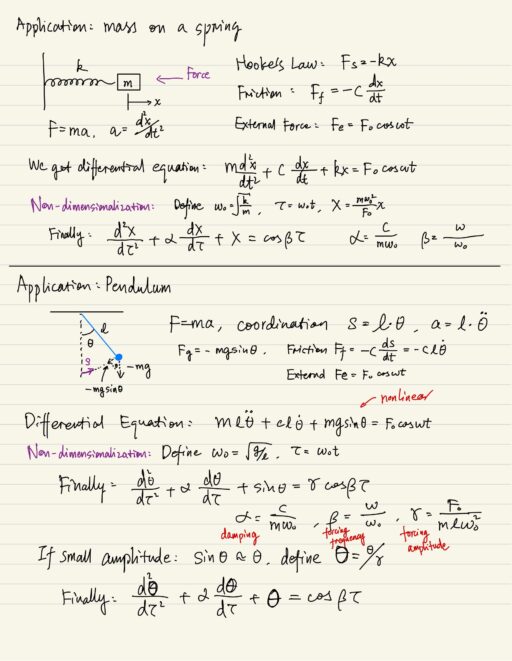
Mass on a spring
You will get an non-dimensionalization equation which is exactly the equation we got for the LRC circuit, except the definitions are different.
Pendulum
Different from previous 2 applications, differential equation in this case is nonlinear. If the oscillation is of a small amplitude, then θ is approximately equal to sinθ. Then you will get an non-dimensionalization equation which is exactly the equation we got for the LRC circuit, except the definitions are different.
Damped Resonance
The three application problems has the same dimensionless differential equation. α presents the damping, which is proportional to the velocity.
x'' + α x' + x = cos βt, α > 0The homogeneous solution to this equation is just a transient. Eventually it will become small and you can neglect it. Final solution will be independent of the initial conditions.
Nondimensionalization
Nondimensionalization is making an equation dimensionless. If we nondimensionalize an equation,it is possible to reduce parameters into a smaller set. The non-dimensional equation here may be equivalent to other physical systems. First you need to know the units of the various things. A physical equation is only valid if every term in that equation has the same units.


My Certificate
For more on Inhomogeneous Linear Differential Equations, please refer to the wonderful course here https://www.coursera.org/learn/differential-equations-engineers
Related Quick Recap
I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai
