Quadrotors: Energetic and System Design

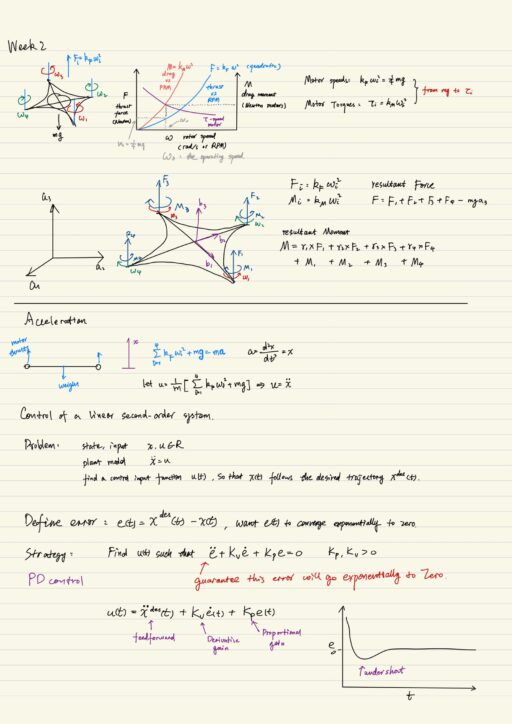
Spinning all rotors of a quad-rotor in the same direction will cause the robot to constantly rotate. The thrust F and drag moment M are quadratic to angular velocity ω. You have to size the motor to product the torque to over come this drag moment. If resultant forces and moments are non-zero, you get acceleration. Vertically, our goal to control this vehicle is to determine the function u ( equals to acceleration a ), such that the vehicle goes to the desired position (x). In this way, control of vertical movement is a second order linear system, it is a Proportional-Derivative (PD) control.

• Proportional control acts like a spring (capacitance) response. The higher the proportional, the more springy the system becomes and more likely it is to overshoot.
• Derivative control is a viscous dashpot (resistance) response. The higher the derivative, the more damped it becomes. Large derivative gain makes the system over-damped and converges slowly (never overshoots).

In more exceptional cases, Proportional-Integral-Derivative control is useful, and the integral term essentially allows you to compensate for unknown effects caused by either unknown quantities, or unknown wind conditions or disturbances. Meanwhile the downside is now the differential equation now becomes third-order.

Motors actually are not capable of producing whatever thrust the controller required. The thrust the motor can produce is limited by the peak torque. The peak torque decides the maximum thrust which decides maximum acceleration. The thrust-weight ratio is important and affects the response of quad-rotor.

When you think about system design, you have to think about battery selection, power consumption, total energy carried by battery. Robot’s hovering is an inefficient mechanism (about 200 Watt/kilo). The smaller you make the vehicle, the larger the acceleration you can produce in the angular direction.

For more on energetic and system design of quad-rotors, please refer to the wonderful course here https://www.coursera.org/learn/robotics-flight/

I am Kesler Zhu, thank you for visiting. Check out all of my course reviews at https://KZHU.ai