Line and Surface Integrals

Line Integrals

Scalar Fields

We have a curve C in the x-y plane, we can represent a point on this curve then by a vector r. To do a line integral, we break the curve into small pieces ds, you have a small element of length ds and a value of f on that element, we multiply them together, and then we sum over all of the elements on the curve C. Then the integral is Riemann sum:

∫c f(r) ds

There are 2 method of solving this integral:

1. Curve is y = y(x)
2. Curve is parameterized x = x(t), y = y(t)

---

---

Vector Fields

Most of the physical problems that we define in terms of line integrals are actually line integrals of a vector field. We have a curve C with direction. On this curve, we have a well-defined vector field u^→ . At the same time we have an element of the curve dr^→, pointing and along the direction of the curve. It’s basically saying that you have to take the component of u^→ along the curve to do the line integral:

∫c u→ ∙ dr→

But this actually can be written in terms of a line integral of a scalar field, where t^ is the tangent unit vector:

∫c (u→ ∙ t^) ds

Usually we do the integral by parameterizing the curve.

Work-Energy Theorem

Work in a physicist definition is the energy transferred to an object by a force. The work is actually line integral of the force along the curve, it is done by the force on the mass results in the change in the kinetic energy of the mass.

Surface Integrals

Scalar Fields

We have some surface in three-dimensions. The surface itself is a two-dimensional object. We have a scalar field f defined on the surface. The surface integral of the scalar field will be obtained by a Riemann sum, where we break the surface into small surface elements dS. Then multiply each element dS by the average value of the scalar field f, and finally we sum over all of those surface elements.

∫S f(r) dS

We compute this integral by parameterizing the surface, by actually parameterizing r. If the scalar field f(r) = 1, then the surface integral just gives us the area of the surface.

Vector Fields

We have a vector field u^→ defined on the surface, the surface integral of the vector field is defined as:

∫S u→ ∙ dS→

It can also be written in terms of a surface integral of a scalar field, where n^ is the normal vector to the surface. The key here is that to define the surface integral of a vector field, we take the dot product of the vector field with the normal unit vector to the surface 

∫S (u→ ∙ n^) dS

Flux Integrals

The surface integral of a vector field is sometimes called a flux integral and the flux integral usually has some physical meaning.

The mass flux is then as the fluid flows through the surface.

∫S ρ u→ ∙ dS→

In the context of electricity and magnetism, we have both electric flux and magnetic flux:

∫S E→ ∙ dS→
∫S B→ ∙ dS→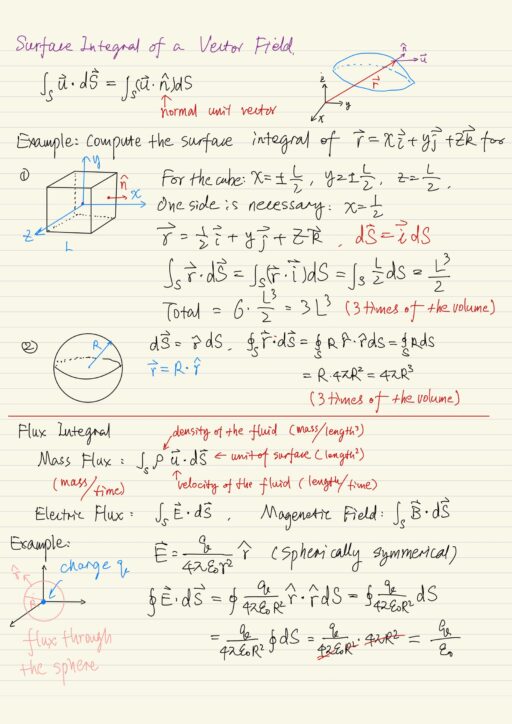

---

---

My Certificate

For more on Line and Surface Integrals, please refer to the wonderful course here https://www.coursera.org/learn/vector-calculus-engineers

My #81 course certificate from Coursera

---

Related Quick Recap

Polar, Cylindrical, Spherical Coordinates

---

I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai