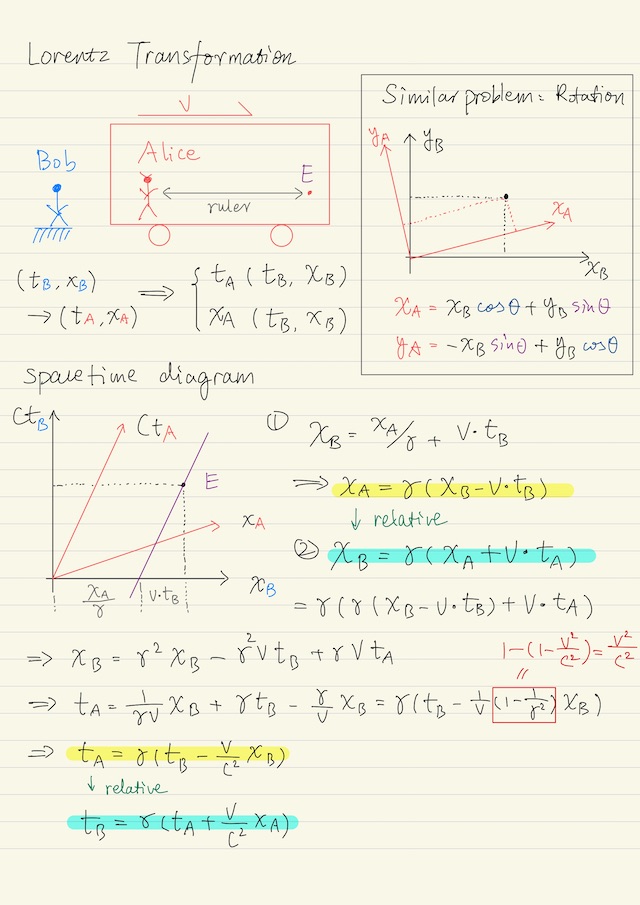
Lorentz transformation is the central question in the mathematical structure of special relativity. Simply put, given the coordinate of the event in one frame, say Bob’s frame, (tB,xB), calculate the coordinate in another frame, say Alice’s frame (tA,xA). Let us first examine a similar problem, which is the rotation of the space directions.
In the problem of Rotation, two frames share the same origin, but there is an angle θ between their axis. The conversion of coordinates from one frame to another frame is:
xA = xB cosθ + yB sinθ
yA = -xB sinθ + yB cosθDerive the Lorentz transformation is similar but in spacetime diagram.
Space Part
Assuming a scenario that Alice is moving in a car, at a particular time there is an event happening in the car at some distance away from Alice. Bob is on the ground, suppose we know that time and space coordinates of this event in the Bob’s frame (tB, xB), then we want to calculate the coordinates in Alice’s frame (tA, xA) as function of (tB, xB). Note xB actually contains two parts:
xA / γ | Length of ruler in Alice’s frame, but also contracted in Bob’s frame because of the speed v. |
tB ∙ v | The distance that the ruler has moved. |
Then we are able to get the first equation of the Lorentz transformation:
xB = xA / γ + v ∙ tB
⟹ xA = γ (xB - v ∙ tB) <--- (1)Time Part
Motion is relative. xB will have a similar equation, but with a speed of different sign.
xB = γ (xA + v ∙ tA) <--- (2)
⟹ xB = γ ( γ (xB - v ∙ tB) + v ∙ tA)Together with xA we’ve got above, we could derive the function tA(tB, xB):
tA = γ (tB - v/c2 xB) <--- (3)Again according to the relativity, we have
tB = γ (tA + v/c2 xA) <--- (4)Total, we’ve got 4 equations, they are equations of Lorentz transformation.
Lorentz Transformation
Lorentz found this a few years before Einstein has discovered the special relativity. Lorentz transformation is formally defined as below, where β = v / c, γ = 1 / √(1 - v2/c2), and the perpendicular y and z directions are included for completeness, which are unchanged during motion.
| Lorentz transformation | Inverse Lorentz transformation |
c tA = γ (c tB - β xB) | c tB = γ (c tA + β xA) |
The Lorentz transformation is the mathematical structure of special relativity. All of the effects, such as same time, time dilation, and length contraction, can be directly read off from these equations.
How to Interpret Same Time
Suppose there are two events of the same time in Alice’s frame. Let ∆tA be the separation of these two events in our Alice’s frame. According to the first equation, we have:
c ∆tA = γ (c ∆tB - β ∆xB) = 0
⟹ tB ≠ 0 As long as there is motion β ≠ 0, the two events are spatially separated ΔxB ≠ 0, then ΔtB is nonzero, meaning that these two events are not of the same time in Bob’s frame. So from Lorentz transformation, we directly derived that the concept of the same time is relative.
How to Interpret Time Dilation
In time dilation, Alice carries a clock, which is actually moving together with Alice. It means the spatial coordinate of the clock is 0 in Alice’s frame xA = 0. Using the first equation of Inverse Lorentz transformation:
c tB = γ (c tA + β xA), and xA = 0
⟹ tB = γ tA How to Interpret Length Contraction
Alice is moving and carrying a ruler. There is a key observation that for a moving ruler, we have to measure the one end and the other end at the same time. Let us just simply take tB = 0, then by using the second equation of Lorentz transform, we have:
xA = γ (xB - β c tB), and tB = 0
⟹ xA = γ xB
⟹ xB = xA / γ Velocity Addition
We could obtain velocity addition rule from Lorentz transformation. Suppose in 3-dimensional space, there is a car moving with velocity v in the x direction, i.e. (v, 0, 0). On the car, there are Alice and another object moving with general velocity (vAx, vAy, vAz) = (dxA/dtA, dyA/dtA, dzA/dtA), where A stands for Alice.
Bob standing on the ground, seeing added velocity:
- the motion of the car.
- the object moving in the car.
Now the question is what is the velocity of the object with respect to Bob (vBx, vBy, vBz)?
vBx = (vAx + v) / (1 + v ∙ vAx / c2)
vBy = (vAy) / γ ∙ (1 + v ∙ vAx / c2)
vBz = (vAz) / γ ∙ (1 + v ∙ vAx / c2)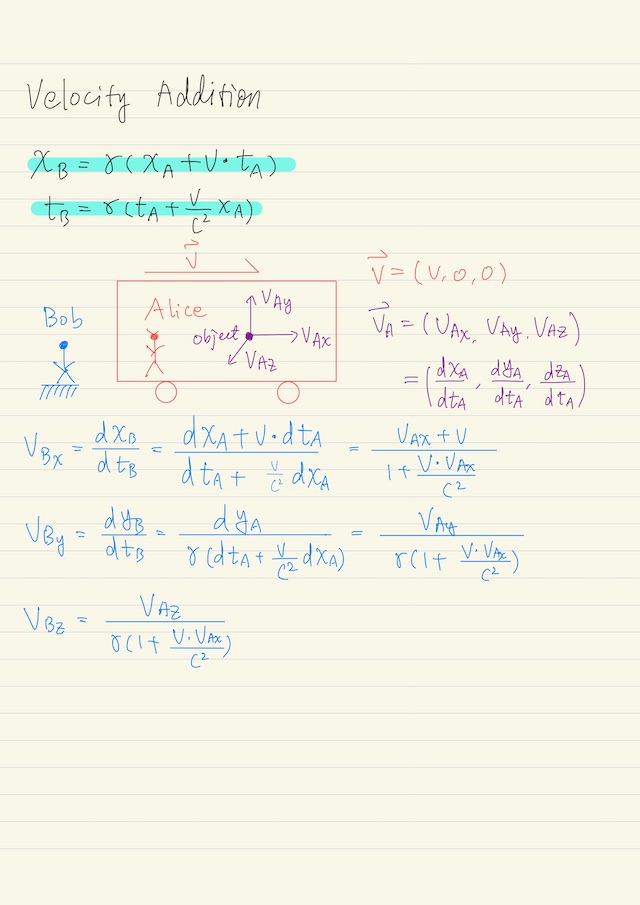
No matter in which direction (x, y, or z), as long as one of the speed goes to the speed of light, all the result that you are getting has the magnitude c. In other words, if you have an object which is moving with speed of light in one frame, it will be the speed of light, in another frame. The components in x, y, z may not be c but the magnitude is c. This is the velocity addition rule.
My Certificate
For more on Lorentz Transformation, please refer to the wonderful course here https://www.coursera.org/learn/understanding-modern-physics-1-relativity-and-cosmology
Related Quick Recap
I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai
Don't forget to sign up newsletter, don't miss any chance to learn.
Or share what you've learned with friends!
Tweet
