Macroscopic Thermodynamics

Table of Contents

First of all, a quick introduction to 4 the most important figures in thermodynamics:

Sadi Carnot (1796-1832) who discovered the second law of thermodynamics.
Rudolf Clausius (1822-1888) who restated the Sadi Carnot’s principle into the form we use today.
William Thomson (Lord Kelvin, 1824-1907) who brought thermodynamics into its modern form.
Max Planck (1858-1947) who is best known for the Planck Function of radiation, heat transfer and as a founder of quantum mechanics.

Essentially all thermodynamic system problems can be cast in a similar form: an isolated system, divided into 2 subsystems by a piston. The question arises when what happen when the piston is:

adiabatic, fixed, and impermeable
diathermal (heat transfer is allowed)
allowed to move
porous to one or more chemical species

Postulatory Basis

The postulates should be the simplest ones that are proved a posteriori and will do the job, rather than be derived by an a priori proof.

a priori	deduction, based on theory (Theory → Hypothesis → Observation → Confirmation)
a posteriori	induction, based on experiment (Observation → Pattern → Tentative Hypothesis → Theory)
ab initio	from the beginning
ipso facto	by the fact

Postulate 1

There exists certain states (equilibrium states) of simple systems that, macroscopically, are characterized completely by the internal energy U, the volume V, and the more numbers N₁, N₂, …, N_r, of the chemical components, where r is the number of chemical components.

The implications of this postulate:

Previous history plays no role in determining the final state. When systems are in equilibrium, the property functions do not depend on any dynamic quantities.
All microscopic quantum states are allowed (a priori probability).
There are violations of this postulate: heat treated steel, non-Newtonian fluid with hysteresis, ortho- and para- hydrogen.

Postulate 2

There exists a function called the entropy S, of the extensive parameters of any composite system, defined for all equilibrium states and having the property that: the values assumed by the extensive parameters are those that maximize the entropy over the manifold of constrained equilibrium states.

This postulate introduces the concept of entropy and essentially states the second law of thermodynamics.

Extensive properties	Those directly depend on the size (total extent) of the system. For example: internal energy U, total volume V, total mass M or moles N, total entropy S, enthalpy H, etc.
Intensive properties	Normalized or not dependent on system size. For example: temperature T, pressure p, chemical potential μ, etc.
Normalized properties	Extensive properties normalized on mass, moles or volume. For example: internal energy u, enthalpy h, entropy s.

Postulate 2 only applies for equilibrium states. Also the total entropy (including all subsystems and surrounding) is maximized. We can solve the Fundamental Problem if we know the Fundamental Relation:

S = S(U, V, N_i)

Examples of Fundamental Relations include Van der Waal’s Liquid, Ideal Gas, etc.

Postulate 3

The entropy of a composite system is additive over the constituent subsystems. The entropy is continuous and differentiable and is a monotonically increasing function of energy.

This postulate simplifies the mathematics: the entropy of the overall system is the sum of the entropies of each subsystem, i.e. S = ∑_j S_j. Next, entropy of each subsystem is a function of the properties of that subsystem only S_j = S_j(U_j, V_j, N_i,j). Third, entropy is a 1st-order function of the extensive properties, i.e. S(λU, λV, λN_i) = λS(U, V, N_i).

The partial derivative of entropy is always positive ∂S/∂U > 0, which means entropy can be inverted with respect to energy in the fundamental equation, U = U(S, V, N_i). Lastly, the extensive properties can be normalized: u ≡ U/N, v ≡ V/N, s ≡ S/N, further more we have

S = N S(U/N, V/N, N_i/N)
s = s(u, v, y_i) # y_i is the mole fraction

Postulate 4 (3rd Law of Thermodynamics)

The entropy of any system vanishes in the state for which ∂S/∂U = 0.

This implies entropy goes to zero as temperature goes to zero.

Temperature

Consider an isolated system composed of 2 subsystems A and B which are initially at different equilibrium states. At the beginning, the barrier between the 2 subsystems was fixed, insulated and impermeable, and nothing could happen. ONLY heat transfer is then allowed across the barrier. If there is a difference in temperature, the heat transfer will occur. But how much?

The way to answer is to find the final equilibrium state, and use the first law to calculate the heat transfer that took place between the initial and final states. The postulates give the rule for finding an equilibrium state. Assuming there is a mixture of species, taking the differential of S gives us:

dS = ∂S/∂U dU + ∂S/∂V dV + ∑_j ∂S/∂N_j dN_j

If the volume V and composition N_j are fixed, then only the first partial derivative will have a value. The total entropy S = S_A + S_B. Differential of S now is:

dS = dS_A + dS_B
⟹ dS = ∂S_A/∂U_A dU_A + ∂S_B/∂U_B dU_B

Now note the energy within the overall system must be conserved U = U_A + U_B, so that dU = dU_A + dU_B = 0, and –dU_A = dU_B. Then eliminate dU_B in dS and set dS to zero.

dS = [∂S_A/∂U_A - ∂S_B/∂U_B] dU_A = 0
⟹ [∂S_A/∂U_A - ∂S_B/∂U_B] = 0
⟹ ∂S_A/∂U_A = ∂S_B/∂U_B
⟹ ∂U_A/∂S_A = ∂U_B/∂S_B ≡ T
⟹ T_A = T_B

The standard thermodynamic definition of temperature T is the partial derivative of internal energy U with respect to entropy S, i.e. T = ∂U/∂S.

Pressure

Both heat transfer across the barrier and motion of barrier are then allowed. If without heat transfer, there is no mechanism to stop the barrier (piston) from oscillating back and forth forever. It is the friction that slows down the piston at its equilibrium position. Friction generates heat, and thus heat transfer.

So now only N_i is fixed, and U and V change. The differential form of Fundamental Relation is:

dS = dS_A + dS_B
⟹ dS = ∂S_A/∂U_A dU_A + ∂S_A/∂V_A dV_A + ∂S_B/∂U_B dU_B + ∂S_B/∂V_B dV_B

Now note the total volume is fixed V = V_A + V_B, so that dV = dV_A + dV_B = 0, and –dV_A = dV_B. Then together with temperature, we eliminate dU_B and dV_B in dS and set dS to zero.

dS = [∂S_A/∂U_A - ∂S_B/∂U_B] dU_A + [∂S_A/∂V_A - ∂S_B/∂V_B] dV_A = 0
⟹ [∂S_A/∂U_A - ∂S_B/∂U_B] = 0 and [∂S_A/∂V_A - ∂S_B/∂V_B] = 0
⟹ ∂S_A/∂U_A = ∂S_B/∂U_B and ∂S_A/∂V_A = ∂S_B/∂V_B
⟹ ∂U_A/∂S_A = ∂U_B/∂S_B ≡ T and ∂S_A/∂V_A = ∂S_B/∂V_B ≡ p/T
⟹ T_A = T_B and p_A = p_B

The standard thermodynamic definition of pressure p is temperature T times the partial derivative of entropy S with respect to volume V, i.e. p = T ∙ ∂S/∂V.

Chemical Potential

Chemical potential plays exactly the same role for mass transfer, as temperature and pressure do for heat transfer and volume change or work.

Consider an isolated system composed of 2 subsystems A and B which are initially at different equilibrium states. At the beginning, the barrier between the 2 subsystems was fixed, insulated and impermeable, and nothing could happen. Both heat transfer and mass transfer across the barrier are allowed.

Assume we have only one species, so i = 1. Now only N₁ and U change. The differential form of Fundamental Relation is:

dS = dS_A + dS_B
⟹ dS = ∂S_A/∂U_A dU_A + ∂S_A/∂N_1,A dN_1,A + ∂S_B/∂U_B dU_B + ∂S_B/∂N_1,B dN_1,B

Now note the total mass is fixed N₁ = N_1,A + N_1,B, so that dN₁ = dN_1,A + dN_1,B = 0, and –dN_1,A = dN_1,B. Then together with temperature, we eliminate dU_B and dN_1,B in dS and set dS to zero.

dS = [∂S_A/∂U_A - ∂S_B/∂U_B] dU_A + [∂S_A/∂N_1,A - ∂S_B/∂N_1,B] dN_1,A = 0
⟹ [∂S_A/∂U_A - ∂S_B/∂U_B] = 0 and [∂S_A/∂N_1,A - ∂S_B/∂N_1,B] = 0
⟹ ∂S_A/∂U_A = ∂S_B/∂U_B and ∂S_A/∂N_1,A = ∂S_B/∂N_1,B
⟹ ∂U_A/∂S_A = ∂U_B/∂S_B ≡ T and ∂S_A/∂N_1,A = ∂S_B/∂N_1,B ≡ -μ/T
⟹ T_A = T_B and μ_1,A = μ_1,B

μ is called chemical potential. Note the minus sign, i.e. μ = -T ∙ ∂S/∂N, because we want the mass transfer to go from the region of higher chemical potential to lower chemical potential.

This concept applies to chemically reacting mixture as well. For a fixed volume V and energy U, this is one equations with r unknowns, if there are r species:

dS = ∑^r_i=1 μ_i/T dN_i = 0

If we take atom conservation into account, then it turns out there are enough equations for the number of unknowns as long as we know the initial condition.

p_k = ∑^r_i=1 n_ki N_i

Representations

By representation, we mean the division of properties: the relations between dependent and independent. For example, in the case of the simple compressible substance, only 2 independent properties are needed to determine all the other properties, say U = U(p, V), or U = U(S, p). Each choice of the two independent properties is a different representation.

We have introduced the Fundamental Relation S = S(U, V, N_i), and its differential form is called Entropy Representation:

dS = 1/T dU + p/T dV - ∑^r_i=1 μ_i/T dN_i

However because of the Postulate 3, we can also write U = U(S, V, N_i), and its differential form is called Energy Representation:

dU = T dS - p dV + ∑^r_i=1 μ_i dN_i

Entropy representation and energy representation contain the same information.

There are as many representation as there are possible combinations of independent variables. The problem is how to retain all the information in the Fundamental Relation when transforming between independent and dependent properties.

Equations of State

In both of the entropy representation and energy representation, the coefficients of the differential form are intensive parameters (T, p, μ) which can be calculated as a function of the independent properties (S, V, N_i) given the Fundamental Relation, i.e.:

T = T(S, V, N_i)
p = p(S, V, N_i)
μ = μ(S, V, N_i)

These relationships are formally called Equations of State. If the Equations of State are known, they can be integrated to recover the Fundamental Relation. This is true in any representation.

Euler Equation

From the definition of the homogenous first-order property, the Fundamental Relation could be written in a convenient form: U(λS, λV, λN) = λU(S, V, N), which means multiplying the independent variable S, V, N with a constant λ, results in the dependent variable U being multiplied by the same factor.

Differentiating both side of the equation with regard to λ, we obtain this expression:

∂U/∂(λS) S + ∂U/∂(λV) V + ∂U/∂(λN) N = U(S, V, N)

Select λ = 1, we got Euler Equation:

U = T S - p V + ∑^r_i=1 μ_i N_i
⟹ S = 1/T U + p/T V - ∑^r_i=1 μ_i/T N_i

Euler Equation provides explicit relationship between the intensive and extensive properties.

Gibbs-Duhem Relation

If we differentiate the Euler Equation and using the differential form of the Fundamental Relation, we derive the Gibbs-Duhem Relation, which shows not all the intensive properties are independent of each other:

S dT - V dp + ∑^r_i=1 N_i dμ_i = 0

The actual number of independent intensive parameters is called the Thermodynamic Degrees of Freedom. A simple system of r components has r+1 degrees of freedom. This corresponds directly to Gibbs Phase Rule.

Alternative Representations

It is often useful to have one or more of the intensive properties as independent. The Legendre transform allows transforming between extensive and intensive properties so that the transform relation contains all the information in the Fundamental Relation.

Various transforms from the energy representation are called Thermodynamic Potentials, because differences in their value can drive action.

Various transforms from the entropy representation are called Massieu Functions.

Extreme Principle: entropy and its transformation are maximized; and energy and its transformation are minimized.

Quasi-Static Process

A quasi-static process is one that occurs slowly enough so that the system appears to be in equilibrium at all times. The practical consequence is that the Fundamental Relation applies during the process. This is an idealization that will be very useful in calculating limiting behavior. It leads to the concept of reservoirs.

Reservoirs are useful idealizations for the way systems interact with their surroundings. We usually make some idealized assumptions about them that leads to simplification. Two commonly used are:

mechanical energy reservoir	Idealization of a very large mechanical sink or source (a giant piston cylinder arrangement). The properties of the reservoir are unchanged even through work is done on / by it. Consider an infinitesimal amount of work dW, then MER is isentropic. `dU = dW = -pdV ⟹ 1/T dU + p/T dV = 0 = dS`
thermal energy reservoir	Idealization of a very large volume whose properties are essentially unaffected when heat is added or subtracted. Consider an infinitesimal amount of heat transfer dQ, then TER is NOT isentropic. `dU = dQ ⟹ 1/T dU = 1/T dQ = dS`

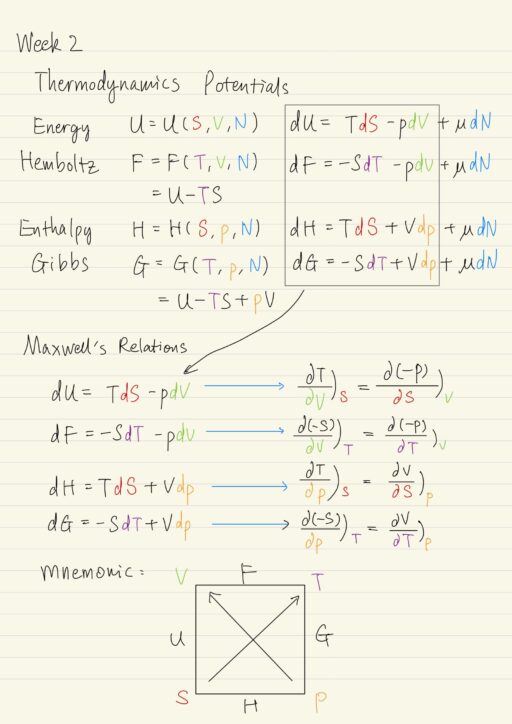
Maxwell’s Relations

James Clerk Maxwell was a 19th century mathematical physicist. Besides Maxwell’s equations of classical electromagnetism, Maxwell also worked on thermodynamics. If we start with the differential form of various thermodynamic potentials, it leads to Maxwell’s Relations.

Building Property Relations

We seek the properties as a function of various independent variables. If we know the Fundamental Relation, then we can use the Legendre transforms and intensive properties definitions to obtain any relationships we might need. Alternatively, we often have experimental data or theoretical results in a particular functional form but need properties in a different form. Traditionally, the experimental approach was to measure the p-V-T relationship and the specific heat.

My Certificate

For more on Macroscopic Thermodynamics, please refer to the wonderful course here https://www.coursera.org/learn/macroscopic-microscopic-thermodynamics

My #86 course certificate from Coursera

Thermodynamics: Fundamental Concepts

I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai

All of your support will be used for maintenance of this site and more great content. I am humbled and grateful for your generosity. Thank you!

Your generosity and kindness is overwhelming!

Don't forget to sign up newsletter, don't miss any chance to learn.

Or share what you've learned with friends!