Vector Calculus: Fundamental Theorems

Gradient Theorem

The fundamental theorem of single variable calculus was the one that told you “the integral of the derivative of a function is just going to be the function itself.”

∫ab f(t) dt = F(b) - F(a)

---

---

The gradient theorem is a generalization of the fundamental theorem of calculus to vector calculus, i.e. calculus of several variables. Suppose a scalar field φ, we could use the gradient of φ to calculate the differential of φ, so the change in φ is equal to the dot product of the gradient of φ with dr.

dφ = ∇φ ∙ dr

If we take line integral of ∇φ ∙ dr along a curve from point r₁ to r₂, we could get the fundamental theorem extended to multi-variables, it tells us that we can do this line integral of a gradient of a function dot product dr, by just knowing the value of the function itself at the endpoints of the curve, just like the fundamental theorem of single-variable calculus.

∫C ∇φ ∙ dr = ∫C dφ = φ(r2) - φ(r1)

If the endpoints are at the same point in space, we’re integrating over a closed curve of ∇φ ∙ dr, then it will be 0.

∮C ∇φ ∙ dr = 0

Conservative Vector Fields

The gradient theorem is closely related to conservative vector fields. Assume u = ∇φ, where φ in physics would be related to the potential energy, and u would be related to the field that the potential energy is arising from. A conservative vector field will be a vector field that satisfies one of four conditions:

u = ∇Φ	∇ × u = 0
∫C ∇φ ∙ dr is path independent	∮C ∇φ ∙ dr = 0

Conservation of Energy

The concept of a conservative vector field is closely related to the physics concept of conservation of energy. Given the work-energy theorem, we know the work done by a force on a mass imports energy to the mass.

Also, conservation of energy occurs when the force is a conservative vector field, by using gradient theorem we end up with the expression of the conservation of energy, where initial kinetic energy T₀ plus initial potential energy V₀ equals to the final kinetic energy T_f plus final potential energy V_f.

T0 + V0 = Tf + Vf

Divergence Theorem

Divergence Theorem is another fundamental theorem of vector calculus. It tells you that a volume integral over the divergence of a vector field is equal to the surface integral over the surface that encloses that volume of the vector field dotted product with ds. It’s reducing a triple integral here, which is a volume integral, into a double integral, which is a surface integral.

∫V ∇ ∙ u dV = ∮S u ∙ dS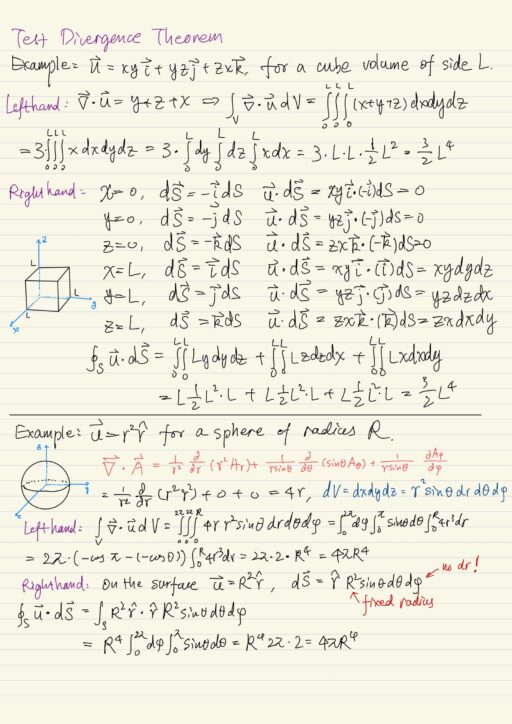

---

---

Continuity Equation for Fluid Flow

It’s a nice theoretical application of the divergence theorem. The fluid itself is continuous. Fluid can be transported from one location in space to another. It’s density can change but it has to do that continuously. We need two quantities:

1. fluid density (mass / unit volume): ρ = ρ(r^→ , t)
2. fluid velocity (length / unit time): u^→ = u^→(r^→, t)

We also need a test volume V with surface S. We try to figure out how the mass M of the fluid in this test volume changes in time: dM/dt, i.e. the derivative of the mass inside this test volume as a derivative with respect to time, which is equal to negative of the mass flux through the surface.

dM/dt = - ⨙S ρ u→ ∙ dS→

This lead to the standard form of continuity equation of fluid flow:

∂ρ/∂t + ∇→ ∙ (ρ u→) = 0

In a special case, where ρ is constant (incompressible fluid) the equation becomes ∇→ ∙ u→ = 0. It means whatever flow into the test volume, has to flow out.

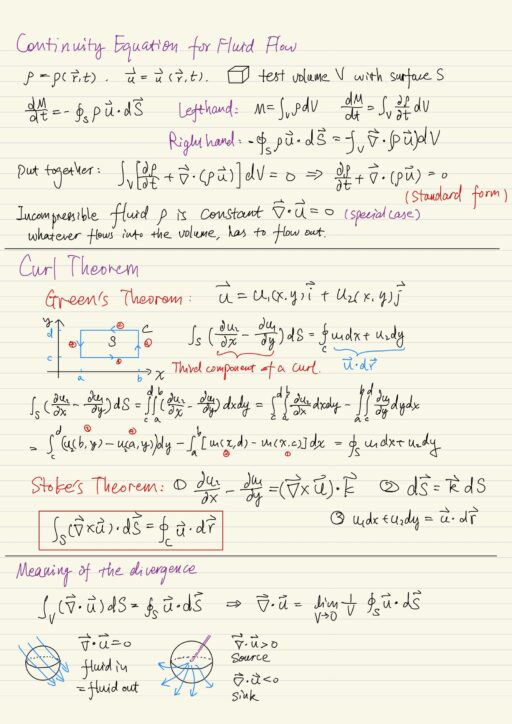
Curl Theorem

Green’s theorem is essentially Stokes’ theorem in two dimensions. Green’s theorem says the integral over an area or a surface in the x, y plane is equal to a line integral enclosing this surface.

∫S (∂u2/∂x - ∂u1/∂y) dS = ∲C u1dx + u2dy

The generalization of Greens’ theorem from 2 dimensions to 3 dimensions gives us the Stoke’s theorem.

∫S (∇→ × u→) ∙ dS→ = ∮C u→ ∙ dr→

Maxwell’s Equation

Divergence Theorem and Stokes Theorem can be used to convert the Maxwell’s equations from the integral form into their differential form.

---

---

My Certificate

For more on Vector Calculus: Fundamental Theorems, please refer to the wonderful course here https://www.coursera.org/learn/vector-calculus-engineers

My #81 course certificate from Coursera

---

Related Quick Recap

Line and Surface Integrals

---

I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai