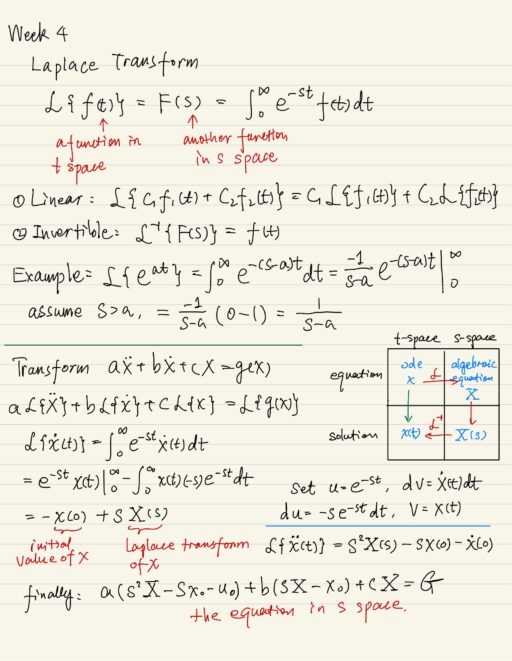
Laplace transform
Laplace transform is a technique for solving differential equations. By using the Laplace transform you can convert a differential equation into another space where the equation is easier to solve. Laplace transform is a linear transformation and it is invertible.
The strength of the Laplace transform technique, is to solve the differential equations where you have a discontinuous right-hand side. So, either the form of the function changes at some time T, or you have an impulse force on the right-hand side.

Heaviside step function
Heaviside step function is used to model discontinuous function, i.e. when the form of the function changes. The uses of Heaviside step function include to shift functions and to glue different functions into one.

The Dirac Delta Function
The Dirac Delta Function is very useful in modeling an impulse force. It can be modeled as limit of a function, using Heaviside step function. Dirac Delta functions makes doing integrals extremely easy.

Series Solution Method
The series solution method is another technique of solving linear differential equations. When all coefficients are constant, you could try an ansatz which is a series solution. Once you substitute ansatz series into the differential equation, next try to write the whole thing as one power series. The theorem there is that if you have a power series equal to 0, then the coefficients of every power of x must be 0.

Airy’s equations y'' - xy = 0
In case there is non-constant coefficient, the “exponential ansatz” y = ert won’t help. The only thing we have available to us here is either a series solution method or a numerical method of solution.

My Certificate
For more on Laplace Transform and Series Solution Method, please refer to the wonderful course here https://www.coursera.org/learn/differential-equations-engineers
Related Quick Recap
I am Kesler Zhu, thank you for visiting my website. Check out more course reviews at https://KZHU.ai